You can also find the answer to these two interesting questions.
- Why does the resistor didn't oppose the current in the series connection?
- Why the current decrease in the parallel connection?
Resistor
The resistor is a linear two-terminal passive component discovered in the year of 1827 by Georg Simon Ohm, a German physicist. SI unit for the electrical resistance is Ohm and its symbol is Ω.
In modern days, all electronic devices use a resistor.
Types of resistor
Resistors are divided into two categories, based on their working type
Fixed resistor
The resistance value will be constant. It cannot be adjusted.
Variable resistor
The resistance value is adjustable. It is possible to change the resistance value according to the application.
Function of resistor in a circuit
The function of a resistor is to oppose the flow of electric current.
R=V/I
We express the opposing value of the resistor in terms of Resistance.
For example, this resistor has a resistance of 50ohm.
Resistor Electrical and electronic symbol
Why does the resistor didn't oppose the current in the series connection?
This is happening to satisfy Kirchhoff's current law, in a closed-loop current entering the (resistor) node is always equal to current leaving the resistor (node).
KVL says that "In a closed-loop voltage drop occurs in a series circuit is always equal to the supply voltage or applied voltage".
Connection of resistor
We can connect the individual resistor in the following ways at a circuit.
- Series connection
- parallel connection
- Series-Parallel Connection
Resistance- series connection
Over one resistor is connected in series on a circuit, as shown in the simple circuit. If we remove the wire between the R1 and R2 end terminal of R1 touches the R2 start terminal.
Characteristic of current and voltage in series connection
The current that flows through all the resistors are equal but the voltage drop across each resistor is different.
Current - Same
Voltage - Difference
The sum of the voltage drop across the individual resistor is equal to the supply voltage.
ie., V= V1+V2----- (1)
The V1 = Voltage drop across resistor 1 (R1)
The V2 = Voltage drop across resistor 2 (R2)
V = power supply
According to Ohm's law
V1 = IR1
V2 =IR2
V =IR1+IR2
To find the total resistance of a circuit, take I commonly outside
V = I (R1 + R2)
V / I = (R1+ R2)
To find, the total resistance (R) in a circuit,
R = R1 + R2
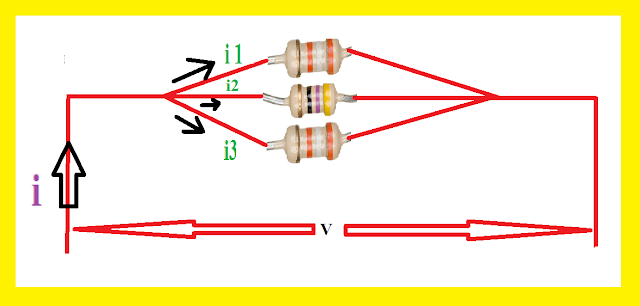
Resistance in parallel connection
Over one resistor connected in parallel where the terminal of the resistors didn't touch by one another.
Characteristic of current and voltage in parallel connection
In this parallel connection, the voltage flow through the individual resistor is the same, but the current flow across each resistor is different.
The voltage across each resistor is equal, and the current across each resistor differ.
Current - Different
Voltage- Same
In the parallel circuit, the sum of the current across an individual resistor is always equal to the supply current.
ie., I = i1+i2+i3
I= Supply current
i1=Current across resistor R1
i2= Current across resistor R2
By ohms law,
i1= V/R1
i2= V/R2
i3= V/R3
therefore substituting V/R1 and V/R2,
I = V/R1 + V/R2 +V/R3
=V (1/R1 + 1/R2 + IR3)
I / V = 1 / R1 + 1 / R2 + 1/R3
Now I / V = R
1 / R= (R2 x R1) / (R1x R2)
To find total resistance (R) in a parallel circuit
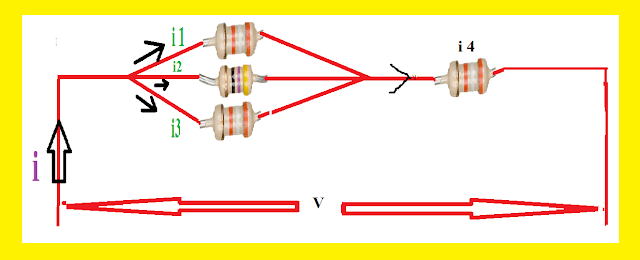
Series-Parallel Connection
In a circuit, we connect some resistors in series, and some resistors are connected in parallel. That connection is called series-parallel connection
Tags:
Basics of Circuit theory